Units conversion by factor-label

Many, if not most, parameters and measurements in the physical sciences and engineering are expressed as a numerical quantity and a corresponding dimensional unit; for example: 1000 kg/m³, 100 kPa/bar, 50 miles per hour, 1000 Btu/lb. Converting from one dimensional unit to another is often somewhat complex and being able to perform such conversions is an important skill to acquire. The factor-label method, also known as the unit-factor method or dimensional analysis, is a widely used approach for performing such conversions.[1][2][3] It is also used for determining whether the two sides of a mathematical equation involving dimensions have the same dimensional units.

Contents

The factor-label method for converting units

The factor-label method is the sequential application of conversion factors expressed as fractions and arranged so that any dimensional unit appearing in both the numerator and denominator of any of the fractions can be cancelled out until only the desired set of dimensional units is obtained. For example, 10 miles per hour can be converted to meters per second by using a sequence of conversion factors as shown below:

10 mile   1609 meter      1 hour           meter
-- ---- × ---- ----- × ---- ------  = 4.47 ------
 1 hour      1 mile    3600 second         second

It can be seen that each conversion factor is equivalent to the value of one. For example, starting with 1 mile = 1609 meters and dividing both sides of the equation by 1 mile yields 1 mile / 1 mile = 1609 meters / 1 mile, which when simplified yields 1 = 1609 meters / 1 mile.

So, when the units mile and hour are cancelled out and the arithmetic is done, 10 miles per hour converts to 4.47 meters per second.

As a more complex example, the concentration of nitrogen oxides (i.e., NOx) in the flue gas from an industrial furnace can be converted to a mass flow rate expressed in grams per hour (i.e., g/h) of NOx by using the following information as shown below:

NOx concentration 
= 10 parts per million by volume = 10 ppmv = 10 volumes/106 volumes
NOx molar mass 
= 46 kg/kgmol (sometimes also expressed as 46 kg/kmol)
Flow rate of flue gas 
= 20 cubic meters per minute = 20 m³/min
The flue gas exits the furnace at 0 °C temperature and 101.325 kPa absolute pressure.
The molar volume of a gas at 0 °C temperature and 101.325 kPa is 22.414 m³/kgmol.
10  m³ NOx   20 m³ gas   60 minute   1      kgmol NOx   46 kg NOx      1000 g          g NOx
--- ------ × -- ------ × -- ------ × ------ --------- × -- --------- × ---- -- = 24.63 -----
106 m³ gas    1 minute    1 hour     22.414 m³ NOx       1 kgmol NOx      1 kg         hour

After cancelling out any dimensional units that appear both in the numerators and denominators of the fractions in the above equation, the NOx concentration of 10 ppmv converts to mass flow rate of 24.63 grams per hour.

Checking equations that involve dimensions

The factor-label method can also be used on any mathematical equation to check whether or not the dimensional units on the left hand side of the equation are the same as the dimensional units on the right hand side of the equation. Having the same units on both sides of an equation does not guarantee that the equation is correct, but having different units on the two sides of an equation does guarantee that the equation is wrong.

For example, check the Universal Gas Law equation of P·V = n·R·T, when:

            mol     (Pa)(m³)     K
(Pa)(m³) = ----- × ---------- × ---
             1      (mol)(K)     1

As can be seen, when the dimensional units appearing in the numerator and denominator of the equation's right hand side are cancelled out, both sides of the equation have the same dimensional units.

Limitations

The factor-label method can convert only unit quantities for which the units are in a linear relationship intersecting at 0. Most units fit this paradigm. An example for which it cannot be used is the conversion between degrees Celsius and kelvins (or Fahrenheit). Between degrees Celsius and kelvins, there is a constant difference rather than a constant ratio, while between Celsius and Fahrenheit there is both a constant difference and a constant ratio. Instead of multiplying the given quantity by a single conversion factor to obtain the converted quantity, it is more logical to think of the original quantity being divided by its unit, being added or subtracted by the constant difference, and the entire operation being multiplied by the new unit. Mathematically, this is an affine transform (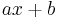ax%2Bb), not a linear transform (ax). Formally, one starts with a displacement (in some units) from one point, and ends with a displacement (in some other units) from some other point.

For instance, the freezing point of water is 0° in Celsius and 32° in Fahrenheit, and a 5° change in Celsius correspond to a 9° change in Fahrenheit. Thus to convert from Fahrenheit to Celsius one subtracts 32° (displacement from one point), multiplies by 5 and divides by 9 (scales by the ratio of units), and adds 0 (displacement from new point). Reversing this yields the formula for Celsius; one could have started with the equivalence between 100° Celsius and 212° Fahrenheit, though this would yield the same formula at the end.

[°F = 1.8(°C) + 32°]

To convert Celsius to Fahrenheit, simply plug in the known numbers in the above formula.

[°C = (°F-32°) ÷ 1.8]

To convert Fahrenheit to Celsius (Centigrade), plug the known temperature into the above formula.

EX. °F = 1.8(-40°C) + 32° = -40°F (Identical temperature point in °C and °F)

EX. °C = (98.6°F-32°) ÷ 1.8 = 37°C (Known standard body temperature in °C and °F)

See also

References

  1. ^ David Goldberg (2006). Fundamentals of Chemistry (5th Edition ed.). McGraw-Hill. ISBN 0-07-322104-X. 
  2. ^ James Ogden (1999). The Handbook of Chemical Engineering. Research & Education Association. ISBN 0-87891-982-1. 
  3. ^ Dimensional Analysis or the Factor Label Method

External links